Architects and construction workers for a new mathematics building calculate the amount of carpeting, wiring, and air conditioning necessary to double the size of the entrance area. A team of radiologists examines a sequence of magnetic resonance images displaying a tumor on a patient's optic nerve as it responds to treatment over the course of a month. A group of geologists studying global warming patterns reconstructs the climate history of the Midwest over ten thousand years. A choreographer challenges her students to dance with their backs flat against a wall. In an interactive computer graphics laboratory, a mathematics professor and her student programmers adapt video game technology for use in the study of complex surfaces. As we will see in the chapters to follow, all of these people shape their experiences by exploiting the concept of dimensions.

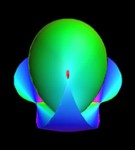
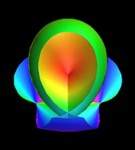
In a modern computer graphics laboratory, it is possible to investigate the interior structure of a complicated mathematical object. These images represent the so-called pedal surface of a curve in three-dimensional space, formed by the closest points to the origin on all planes that just touch the curve at some point. The red spot on the complete figure is called a "swallowtail catastrophe," and the cutaway views show the structure of the surface near such a point.

Although these examples all make use of the notion of dimension, they interpret it differently. The word dimension is used in many ways in ordinary speech, and it has several technical meanings as well. When we refer to a "new dimension," it almost always means that we are measuring some phenomenon along a new direction. The word can be used as a metaphor, as for example when we discover that a rather "one-dimensional" colleague is an accomplished guitarist and skeet shooter, giving her two additional "dimensions of personality." In more conventional usage, dimensions are measurements that can specify location — for example the latitude, longitude, and depth of a submarine — or that can specify shape — perhaps the height, top radius, and bottom radius of a tapering flagpole. A list of dimensions may include other kinds of characteristics, as when we specify a brass gong by its weight, thickness, radius, brightness, and tone. We use time and space together as dimensions when we make an appointment to meet someone at nine o'clock on the corner of Fourth Avenue and Twenty-Third Street on the thirty-seventh floor. In recent years, physicists have begun to speak about configurations that involve 11 or 26 dimensions. Mathematicians often speak about structures in n-dimensional space.

One very common way of thinking of dimensions is to look at what engineers call "degrees of freedom." This notion is implicit in much of our day-to-day activity, as in the following scenario. A driver finds herself in a tunnel under Baltimore harbor crawling behind a large truck. "Do Not Change Lanes," she is admonished. She is stuck in one dimension, effectively kept in line, blocked by the vehicle in front of her and the one in back.

Once outside the tunnel, she is again able to move in two dimensions, because she now has an additional "degree of freedom," allowing her to change lanes to the right or to the left. But a bit later she finds all lanes blocked by bridge construction in Havre de Grace. Trucks and cars hem her in on all sides. She wishes she could escape into that inviting third dimension, where a police helicopter hovers unconstrained by the traffic on the roadway. Her degrees of freedom are not limited to spatial dimensions. She may also wish that she had used another kind of dimension to alleviate her problem, the dimension of time. If only she had timed her trip to arrive at the bridge at a slack period with no traffic buildup.

All of these notions of dimension share some characteristics, which we begin to appreciate better as we try to visualize the relationships they represent.

			Dimensions as Coordinates
			Table of Contents
			Introduction