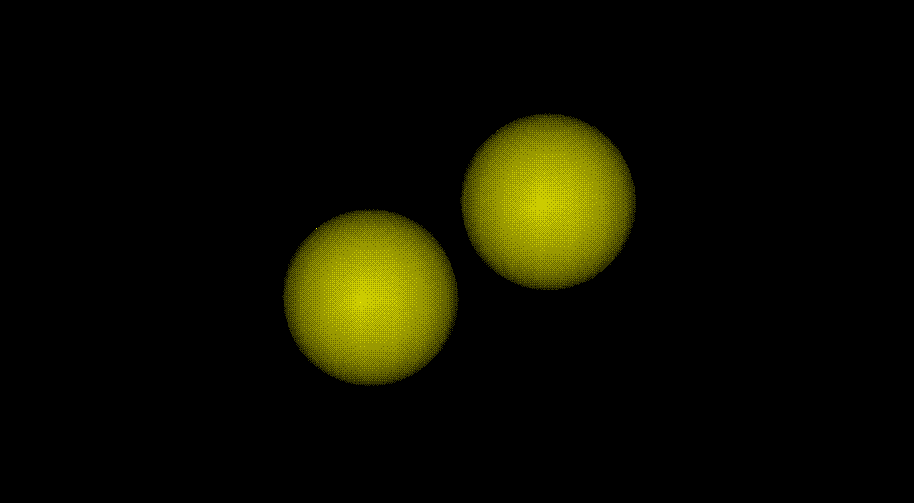Symmetry in Different Dimensions Symmetry in space of One Dimension There isn't any. When there is only a point the entire world is symmetrical. Symmetry in space of two dimensions The two-dimensional place is the place where much thought about symmetry as well as tessellation has been illustrated. Symmetry in two-space is looked at differently depending on what kind of translation you have. Reflections are across lines. Rotations are around points. Symmetry in three-space is more complicated. Rotations are around lines. Reflections are across  planes. If we try to think up to four dimensions, some interesting things come up. Rotation would be around a plane. Reflections might be across cubes. In the fifth dimension, symmetry of rotation would be found across cubes or other solids, and relfective symmetry would appear around hypercubes. Cool, huh? more on  Symmetry in Three Space We have already looked extensively at symmetry in two dimensions.  Here we will examine how the same operations that we used in two space can be used in three space.  Let us look at how we can apply them to this sphere.

 

Translation Translation works just the same way.  We simply change the coordinates of the objects location.  Instead of two variables there are now three.  Everything else about the object is preserved.

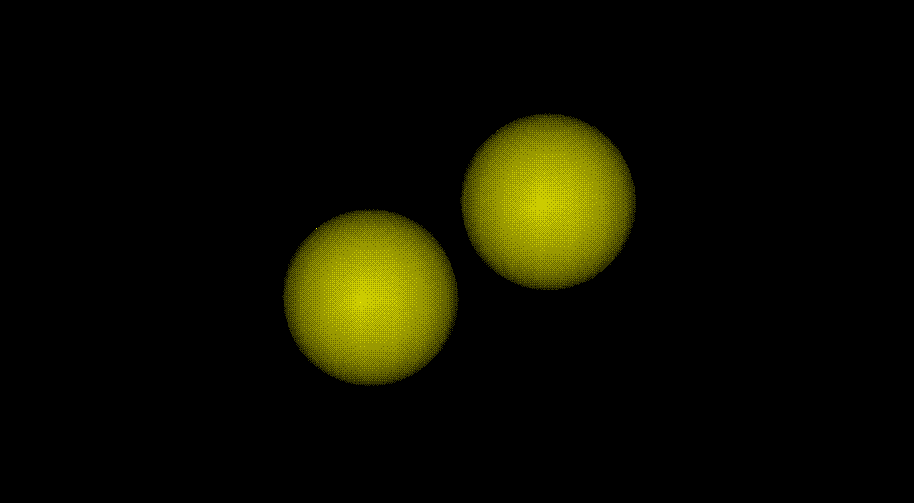
Reflection Reflection works differently in three space.  Instead of reflecting around a line we must reflect along a plane.  If we reflect a sphere along a plane that intersects its center there is no visible difference.  However, if we reflect along a plane that intersects the sphere at a place other than the center we get an object that looks like this.

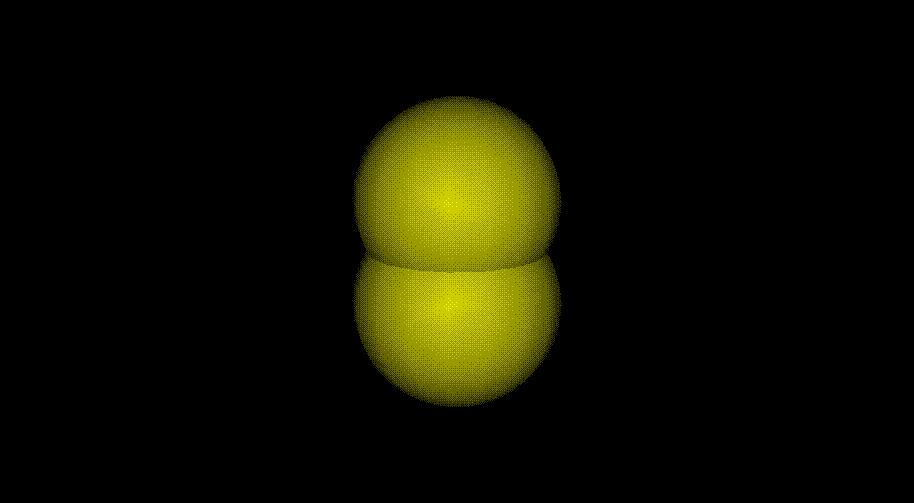
Rotation Once again, rotation is slightly different in three dimensions than in two.  Instead of rotating around a point, we must rotate around a line.  It is possible to rotate an object around a point.  However, what you are really doing is rotating it around one line, and then another.  Again, if the axis of rotation is through the center of a sphere there is no visible difference.  If the axis is through a tangent to the sphere, you get the following sphere.

If you rotate the object around an axis outside of the sphere, you get the following picture.  As you can start to see, if you rotated infinitely many spheres about the same axis, you would get a torus.

home