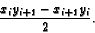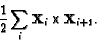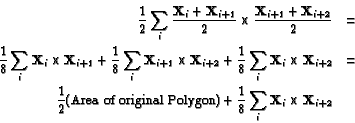Midpoint Polygons

Convex Pentagons

Perimeter

What about perimeters for midpoint pentagons? For a regular pentagon, the midpoint polygon is another regular pentagon with sides equal to one-half the length of each diagonal. The ratio of the midpoint perimeter to the original is then the half the ratio of a side to a diagonal, i.e. one-half the reciprocal of the golden section, = 1/(2t), about .806 The same analysis shows that for a general convex pentagon, the perimeter of the midpoint polygon is half the sum of the lengths of the diagonals, and this definitely is not a constant.

Area

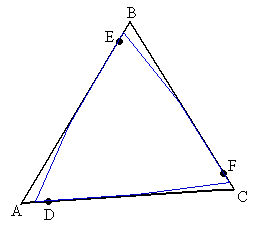
Now what about the areas of midpoint pentagons for convex pentagons? For a regular pentagon, the area of the midpoint pentagon divided by the area of the original figure is 1/(4t²). However for a pentagon close to a triangle, with two vertices close to one vertex on the bottom and two close to the other vertex on the bottom, the area of the midpoint figure is as close to 3/4 as we please. This appears to be the maximum ratio attainable for pentagons.

Demonstration 4. Area of midpoint pentagons

Note at this point, that for convex polygons with six or more sides, the ratio of the area of the midpoint pentagon to the area of the original is as close to 1 as we please. We can achieve this by placing the first two vertices near one vertex of a triangle, the next two near the second, and all the rest near the third. The ratio cannot be larger than 1 since the midpoint polygon lies inside the original convex polygon.

Figure 4. Area ratio approaching 1 for a midpoint hexagon

Surprisingly although the area ratio for a pentagon is not constant, we can still find a relationship between the area of the original, the area of the midpoint polygon, and the area of another figure. This is first seen analytically, and later by a direct geometric argument.

Recall that for a convex polygon with the origin in the interior, we can find the area by adding up the areas of the triangles with the origin as one vertex and a side of the polygon as the opposite sign. If the coordinates of the ith vertex are (x_i,y_i), then the area of the ith triangle, with vertices (0,0),(x_i,y_i), (x_i+1,y_i+1) is

In vector notation, if (x_i,y_i) = X_i, then the area is

The vertices of the midpoint polygon on the other hand are Y_i = (X_i+X_i+1)/2 so the area of the midpoint polygon is

What is the meaning of the additional term? Formally, in the case of a pentagon, it represents 1/4 times the area of the polygon with vertices X₁, X₃, X₅, X₂, X₄. This is the so-called "star pentagon" formed by taking the five diagonals in sequence, a self-intersecting polygon. The sum of the five triangles will give the area of the star, but with the middle pentagon in the star counted twice.

Next: general midpoint polygons
Previous: quadrilaterals