|
Partial Derivatives
Text The partial derivatives of a function of three variables are the slopes of the slice curves.
 If f(x,y,z) is a function of three variables, then for each y = y0 and z = z0, the function f(x,y0,z0) is a differentiable function of x, and its derivative is denoted fx(x0,y0,z0), called the first partial derivative of f with respect to x.
If f(x,y,z) is a function of three variables, then for each y = y0 and z = z0, the function f(x,y0,z0) is a differentiable function of x, and its derivative is denoted fx(x0,y0,z0), called the first partial derivative of f with respect to x.
Similarly the derivative of the x = x0, z = z0 slice curve f(x0,y,z0) with respect to y is denoted by fy(x0,y0,z0), called the first partial derivative of f with respect to y and the derivative of the x = x0, y = y0 slice curve f(x0,y0,z) with respect to z is denoted by fz(x0,y0,z0), called the first partial derivative of f with respect to z.

Demos
Partial Derivatives

| 
|
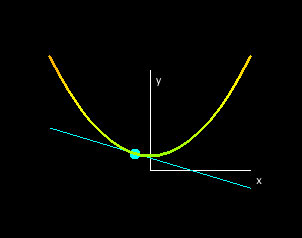
This demo shows the slice curves at a point (P,f(P)), where P is a point in the domain. The slopes of the slice curves at P give the partial derivatives with respect to x, y and z at (P,f(P)). You can use the hotspot in the "Domain" window to get the partial derivatives of every point in the domain. 
|
Exercises 1. Position the hotspot at the origin. What are the slopes of the tangent lines at this point?
2. Where are the slopes of the tangent lines the greatest for all three slice curves?
|