
Visualizing a function can give a mathematician enormous insight into the function's algebraic and geometrical properties. The easiest way to see what a function looks like is to use a computer as a graphing tool. At times, this technique is the most useful, but drawing the function yourself is always the best way to get a feeling for why the function looks the way it does when graphed. All of you are by now familiar with graphing one-variable functions in the plane and hopefully can easily predict the shape of any fairly simple 2D function just by analyzing its equation. This knowledge came from graphing similar functions over and over again to get a feel for their general shape and critical points. The purpose of this tutorial is to quickly revisit these 2D examples and then to move on to drawing functions situated in 3D and even 4D space.

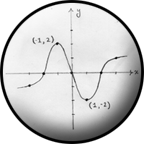

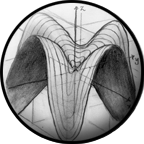

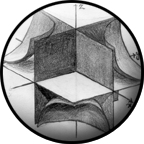


Practice Problems
3D Practice Problems
4D Practice Problems
More Tutorials
I need help with 3D Shading!
I need help with 4D Shading!
I need help with Projections and Mappings!
Why are we using fences???
What size 3D fence should I use?
What size 4D fence should I use?