|
Level Sets & Contour Points
Text The collection of all points x in the domain of a function f for which f(x) = c is called the level set of f at level c.
 The level set of f is empty if there is no point x in the domain of f for which f(x) = c.
The level set of f is empty if there is no point x in the domain of f for which f(x) = c.

In general the contour of a funcion f of one real variable will be a finite set of points in the domain.
Demos
Contour Points

| 
|
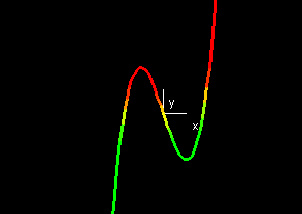
This demo shows the contour points of a function f(x), colored according to the value of f(x). Points that have the same color are part of the same level set. 
|
Exercises 1. Find the points in the level set for each of the following:
- f(x) = x, c = 0
- f(x) = x2 - 1, c = 0
- f(x) = sin(x), c = √2/2
- f(x) = tan(x), c = 1
2. Given a function f(x) with level set A at c = 0 and a function g(x) with level set B at c = 0, what is the level set of the function fg(x) (equal to f(x) * g(x)) at c = 0?
|