« Course List

MA35 Labs

1 » Single Variable Calculus

2 Two Variable Calculus

3 Three Variable Calculus

Polar Coordinate Labs

Two Variable Functions

Contents

1.1 Functions of One Variable

1.2 Differentiation

1.3 More Derivatives

1.3.1 Second Derivatives

1.3.2 Convexity and Concavity

1.3.3 Derivative Test

1.3.4 Taylor Series

1.3.5 Constrained Maxima & Minima

1.3.6 Decomposition of Acceleration

1.4 Integration

1.5 Fundamental Theorem of Calculus

Search

Convexity and Concavity

Text

The second derivative of a function is the derivative of the first derivative. If f'(x) itself is differentiable, then its derivative with respect to x is denoted f''(x). If f''(x) > 0, then f'(x) is increasing and the shape of the graph is concave upward (or convex downward). If f''(x) < 0, then d'(x) is decreasing and the graph is concave downward (or convex upward). A point where the sign of the second derivative changes sign is called and inflection point.

Demos

Convexity and Concavity
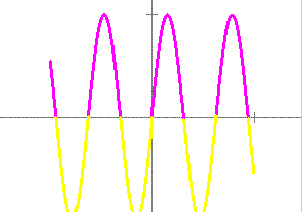
	This demonstration is similar to 1.2.2 (critical points). As in 1.2.2, the function f(x) and its domain are defined in the control panel. This time, the graph of the function is colored magenta where it is concave downward and yellow where it is concave upward.

Exercises