|
Critical Points
(Page: 1
| 2 ) Text A critical point of a parametric surface (x(u,v),y(u,v),z(u,v)) is a point (u0, v0) such that the tangent plane is parallel to the xy, xz, or yz plane.

(u0, v0) will be a critical point of (x(u,v),y(u,v),z(u,v)) if and only if at least one of the following is true:
1. x(u, v) is differentiable and the tangent plane is parallel to the yz plane at (x(u0, v0), y(u0, v0), z(u0, v0)).
2. y(u, v) is differentiable and the tangent plane is parallel to the xz plane at (x(u0, v0), y(u0, v0), z(u0, v0)).
3. z(u, v) is differentiable and the tangent plane is parallel to the xy plane at (x(u0, v0), y(u0, v0), z(u0, v0)).

Demos
Critical Points for Parametric Surfaces

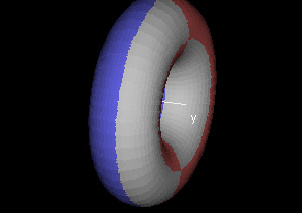
| 
|
This demo shows three graphs of a the same parametric surface (x(u,v), y(u,v), z(u,v)). Each graph is colored according to the signs of the components of the normal vectors to the surface. In the "critical points of z(u, v)" window, a positive x component of the normal vector adds a red layer of color and a positive y component adds a blue layer. Where these overlap, the surface is colored purple. Any point that is on the boundary between a region with red and a region without red and is also on the boundary between a region with blue and a region without blue is a critical point (the significance is that the x and y components of the normal vector are equal to 0 at such a point).
In the "critical points of y(u, v)" graph, a positive z component of the normal vector yields a red layer and a positive x component yields a blue layer.
In the "critical points of x(u, v)" graph, a positive y component of the normal vector yields a red layer and a positive z component yields a blue layer. 
|
Exercises Find the critical points of the following parametrized surfaces:
- (2u, 2v, 1 - u2 - v2), -1 ≤ u ≤ 1, -1 ≤ v ≤ 1
- (cos(u)cos(v), sin(u)cos(v), sin(v)), 0 ≤ u ≤ 2π, -π/2 ≤ v ≤ π/2
- ((2 + cos(v)), sin(v), (2 + cos(v))sin(u)), -π ≤ u ≤ π, -π ≤ v ≤ π
- ((2 + cos(v)), (2 + cos(v))sin(u), sin(v)), -π ≤ u ≤ π, -π ≤ v ≤ π
|