« Course List

MA35 Labs

1 Single Variable Calculus

2 » Two Variable Calculus

3 Three Variable Calculus

Polar Coordinate Labs

Two Variable Functions

Contents

2.1 Functions of Two Variables

2.2 Differentiation

2.3 More Derivatives

2.4 Integration

2.4.1 Volume Under a Function Graph

2.4.2 Riemann Integral

2.4.3 Volume between Function Graphs

2.4.4 Change of Order of Integration

2.4.5 Surface Area for Function Graphs

2.4.6 Change of Variables

2.4.7 Total Mass of a Region in the Plane

2.4.8 Center of Mass

2.4.9 Moment of Inertia

2.5 Vector Analysis

Search

Moment of Inertia

Text

The moment of inertia I of an object about an axis of rotation is a value which indicates the resistance of that object to changes in rotation about that axis. For a region D in the plane with area density ρ(x, y), this calculated using the following formula:

I = ∫(r(x, y))²ρ(x, y)dxdy

Where r(x, y) is the distance of the point (x, y) from the axis of rotation. In the two-dimensional case, this axis is a point.

For an object of density 1, the moment of inertia will be the density multiplied by the integral

I = ∫(r(x, y))²dxdy.

Demos

Moment of Inertia
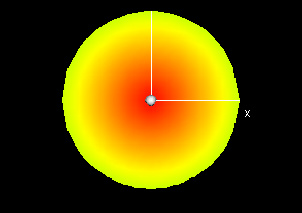
	This demo finds the moment of inertia for a region in the plane of uniform density. The bounds of y are expressed as functions f and g of x. The graph shown displays the region, coloring each point based on its distance from the center of rotation. The closer a point mass is to the center of rotation, the less it adds to the moment of inertia.

Exercises