« Course List

MA35 Labs

1 Single Variable Calculus

2 » Two Variable Calculus

3 Three Variable Calculus

Polar Coordinate Labs

Two Variable Functions

Contents

2.1 Functions of Two Variables

2.2 Differentiation

2.2.1 Partial Derivatives

2.2.2 Critical Points

2.2.3 Tangent Planes and Normal Vectors

2.2.4 Chain Rule

2.2.5 Differentiability

2.3 More Derivatives

2.4 Integration

2.5 Vector Analysis

Search

Tangent Planes and Normal Vectors (Page: 1 | 2 )

Text

If both partial derivatives for each coordinate function of the parametrized surface (x(u,v), y(u,v), z(u,v)) exist at a point (u₀,v₀), then the tangent plane of the surface at (u₀,v₀) is the plane determined by the vectors

(x_u(u₀,v₀), y_u(u₀,v₀), z_u(u₀,v₀)) and

(x_v(u₀,v₀), y_v(u₀,v₀), z_v(u₀,v₀)).

The unit normal to the point (x(u₀,v₀), y(u₀,v₀), z(u₀,v₀)) is the cross product of the two vectors above divided by this cross product's magnitude.

Demos

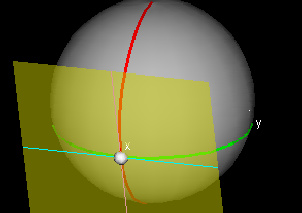
Tangent Plane to a Parametrized Surface

For this demonstration, choose a point (u0,v0) in the domain. You will see a graph of the parametrized surface (x(u,v), y(u,v), z(u,v)) and the u- and v-slice curves at the point (x(u0,v0), y(u0,v0), z(u0,v0)). The tangent lines to each of the slice curves at (x(u0,v0), y(u0,v0), z(u0,v0)) are also drawn, and it is clear that together they determine a plane. This plane, which is shown in yellow, is tangent to the graph at (x(u0,v0), y(u0,v0), z(u0,v0)).

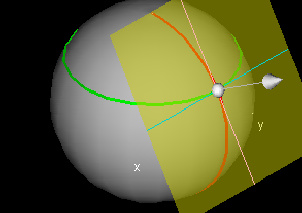
Normal Vector to a Parametrized Surface
	This demo shows the unit normal vector to the tangent plane for a given point (u0,v0) in the domain.