|
Introduction
(Page: Demo Tutorial
| Functions ) Text Calculus is the study of functions.
In the study of functions of
one variable, we encounter domains and ranges of functions, function
graphs, and properties of functions such as continuity. In multivariable
calculus we will extend all of these notions to functions of two and
eventually more variables. We will also deal with functions of one
variable associated with functions of a single variable, namely slice
curves and contour lines.
Demos
Linear Functions
| 
|
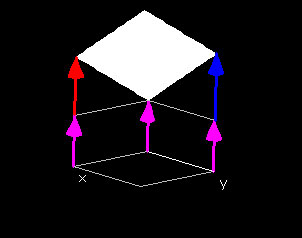
This demo shows the linear function in two variables L(x,y) = px + qy +k over the rectangular domain 0 ≤ x ≤ 1, 0 ≤ y ≤ 1. You can change the constants p,q and k using the respective red, blue or purple slide bar, and the Graph: L(x,y) window will show the function graph of L(x,y) with the chosen values for the constants.
If you slide the hotspot on the purple slidebar, (thereby varying the constant k) the function graph is translated up and down in the directon of f(x,y). Varying p,and r on the red and blue slidebars will scale in the x- and y- direction of the graph.
|
Examples The Zero Function
The simplest function of all is the zero function, defined by f(x,y) = 0 for all x, y. This function is defined for all (x,y) in the plane, and the only value taken on by the function is 0.
Constant Functions
The next simplest class of functions are the constant functions
defined by f(x,y) = k for all x, y. A constant function can be defined for all (x,y) in the plane and the only value taken on by the function is the single point k.
Linear Functions
Linear functions are the next most complicated class of functions, defined
by L(x,y) = px + qy + k. The numbers p and q are called the
x-slope and the y-slope of the linear function and k is called its z-intercept. The linear function
L is defined for all pairs (x,y) of real numbers. If p ≠ 0 or q ≠ 0, then the collection of values L(x,y) will include all real numbers.
Exercises 1. Show that if p ≠ 0, then for every z there is a point (x,y) such that L(x,y) = px + qy + k = z. Show that if p = 0 but q ≠ 0, then the same result holds.
2. Consider the planar triangle with vertices at the x-intercept, y-intercept, and z-intercept points of the graph of L(x,y) = px + qy + k. For what values of p, q, and k does this triangle intersect the graph of L(x,y) over the domain 0 {leq} x {leq} 1, 0 {leq} y {leq} 1?
|