|
Surface Integrals over Function Graphs
Text The surface integral of a function f(x, y, z) over the function graph S of a function g(x, y) for some domain D is defined as follows:
∫∫Sf(x, y, z)dS = ∫∫Df(x, y, g(x, y))√(1 + gx2 + gy2)dxdy

Some applications which illustrate the properties of surface integrals:
When f(x, y, z) = 1, this integral gives the surface area of S.
If f(x, y, z) is a density function, then the surface integral gives the total mass of the surface.

Demos
Surface Integrals over Function Graphs

| 
|
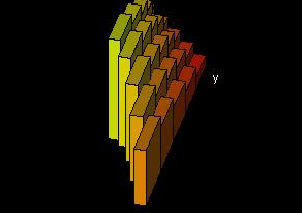
The surface integral of some function f(x, y, z) over the graph of a function g(x, y) is illustrated here. One window shows the "shingles" of g(x, y), colored according to the value of f(x, y, z), and the second displays the value of the integral as the volume above the x-y plane minus the volume below the x-y plane. Note that each rectangular prism in this second window has a base equivalent in area to that of a corresponding shingle in the first window, and a height corresponding to the value of f(x, y, z) for that shingle. 
|
Exercises 1. Find the surface area of the graph of g(x, y) = x2 + y2, 0 ≤ x ≤ 1, 0 ≤ y ≤ 1.
2. Evaluate ∫∫D(x + y + g(x, y))sqrt(1 + gx2 + gy2)dxdy
where g(x, y) = xy and D is defined such that 0 ≤ x ≤ 1, 0 ≤ y ≤ 1.
|